
I sort of dislike this kind of "philosophical" introduction to calculus. From what I understand he’s a computer programmer and math teacher without extensive training in biology I wouldn’t expect him to have any insight into evolutionary theory.
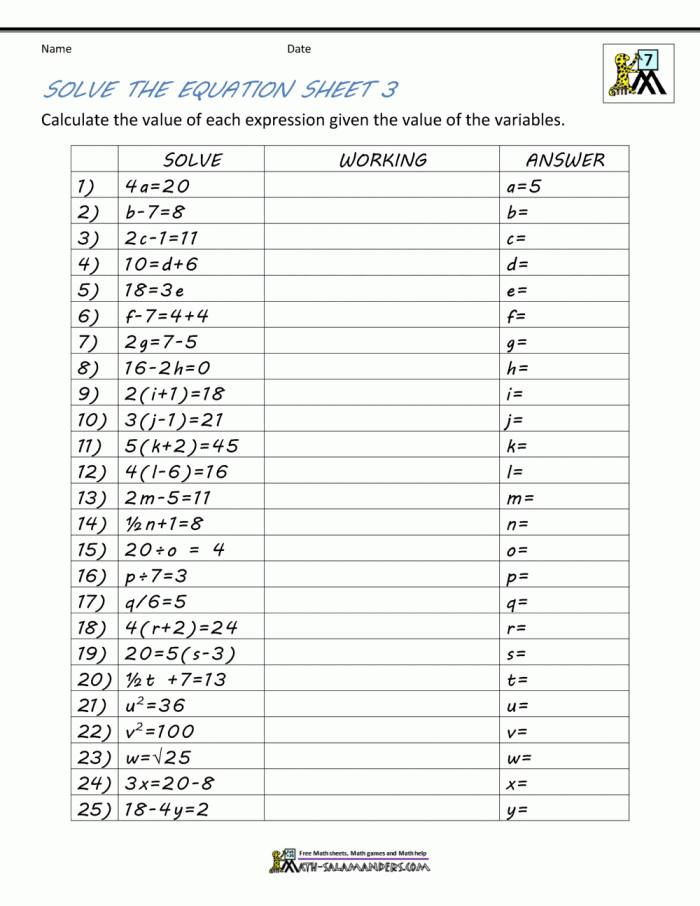
It’s not clear why the guy’s ideas about evolutionary theory (which I know nothing about) have anything to do with his ideas about derivatives. I haven’t ever taught an introductory calculus course, but I think it seems entirely plausible that this approach would save some confusion for many students (this is something which could be tested empirically, if any math-ed researcher has the time and budget for a study). But the pedagogical idea of focusing on differentials seems sound. I certainly wouldn’t recommend this as a sole introductory textbook it’s obviously produced on a shoestring budget (a LaTeX file with the default template sent over to a print shop) and is somewhat limited in many aspects compared to established calculus textbooks.
The book doesn’t really have any technical content that isn’t in those just more elaboration and exercises pitched at students coming to the subject for the first time. You can read the two arxiv papers if you like. Now I'm basically re-doing high school maths. This happened again and again, going lower and lower. It was very difficult, but after heroic efforts, I made breakthroughs! After a while, I noticed these "breakthroughs" were mostly entirely to do with material from lower levels. In my personal journey, I started off with your perspective of just learning the higher levels that I directly needed. There are specifications all over the place.īut my experience with mathematics is completely different - you have to understand the lower level to understand the next level. the JLS defines Java independently of hardware IEEE 754 is similar for fp arithmetic. But if you need to modify techniques, or debug them, it's kind of impossibly frustrating without actually knowing what you're doing!īTW computers have much cleaner abstractions than mathematics. For standard usage, on the "happy path", this is fine. To some extent you're right, the benefit of a higher-level abstraction is using it without knowing the details.


 0 kommentar(er)
0 kommentar(er)
